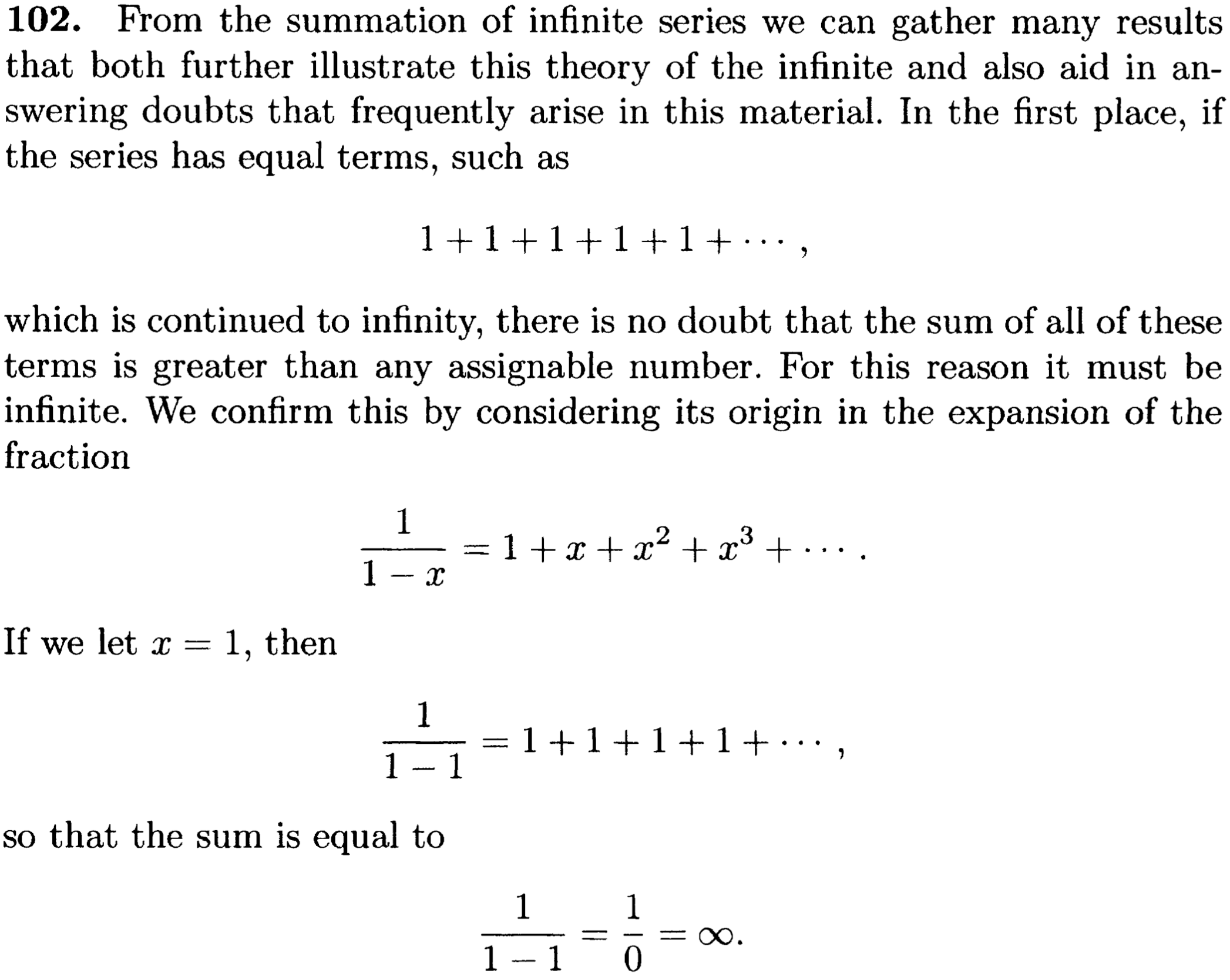
Series(ly)? What is going on?
Consider the following: \[ 1+1+1+1+\cdots \]
What is the value of this infinite sum? It's value is $\infty$!
Let's use the identity: $ \;\;\displaystyle\frac{1}{1-x}= 1+ x+ x^2+ x^3+\cdots $
If we let $x=1$, then $\displaystyle \frac{1}{1-1}$ $= 1+ 1+ 1^2+ 1^3+\cdots$
Thus $ \displaystyle \frac{1}{0} =\infty. $ Wait, whaaat? 😲
Series(ly)? What is going on?
|

Institutiones Calculis Differentialis |
Series(ly)? What is going on?
If we use again this $\;\ds \frac{1}{1-x}= 1+ x+ x^2+ x^3+x^4+\cdots, $
we obtain for example
$-1=$ $\ds \frac{1}{1-2}$ $\displaystyle =1+2+4+8+16\cdots$
$ \ds-\frac{1}{2}=$ $ \displaystyle \frac{1}{1-3} $ $ =1+3+9+27+81\cdots$
$ \displaystyle -\frac{1}{3}=$ $ \displaystyle \frac{1}{1-4} $ $ \displaystyle= 1+4+16+64+256\cdots $
This is counter-intuitive! 🤔
Series(ly)? What is going on?
Now consider the identity $\displaystyle \frac{1}{1+x}= 1-x+ x^2- x^3 + x^4-\cdots,$
we obtain
$\qquad \qquad \displaystyle 1-1+1-1+1-1+\cdots = \frac{1}{1+1} =\frac{1}{2}\;$
$\qquad \qquad \displaystyle 1-2+4-8+16-32+\cdots = \frac{1}{1+2} =\frac{1}{3}\;$
$\qquad \qquad \displaystyle 1-3+9-27+81-243\cdots = \frac{1}{1+3} =\frac{1}{4}.$
So many contradictions! 🤯 😵💫
Series(ly)? What is going on?
|
111. These inconveniences and apparent contradictions can be avoided if we give the word sum [of an infinite series] a meaning different from the usual. 🤔 |

Institutiones Calculis Differentialis |