Multivariate Calculus
&
Ordinary Differential Equations
Lecture 13
1 Ordinary Differential Equations
18.2 The damped spring
Use mouse to drag mass and release.
Compare with the
undamped spring simulation shown before.
Source code
1 Ordinary Differential Equations
18.2 The damped spring
If you try the "spring-weight" system at home it is unlikely to display true simple harmonic motion. If that were possible you could build a perpetuum mobile.
To make the model more realistic we need to take into account the effect of damping. This is caused by air-resistance and mechanical friction of the spring.
1 Ordinary Differential Equations
18.2 The damped spring
The most common model of damping assumes that it corresponds to a force $F_d,$ proportional to the velocity of the moving object, and opposite to the direction of movement. Hence
$F_d =-\beta x',$
where $\beta>0$ is the damping constant measured in $\text{N s m}^{-1}.$
The total force $F$ for the damped spring is thus given by
$F= \underbrace{-\beta x'}_{\text{damping force}}\quad \underbrace{-k(s+x)}_{\text{restoring force}}+ \underbrace{m g}_{\text{gravitational force}}.$
1 Ordinary Differential Equations
18.2 The damped spring
$F= \underbrace{-\beta x'}_{\text{damping force}}\quad \underbrace{-k(s+x)}_{\text{restoring force}}+ \underbrace{m g}_{\text{gravitational force}}.$
As before, $k s=m g,$ so that
$F=-\beta x'-kx.$
Thanks to Newton's second law this yields the equation of motion
$m x''+\beta x'+k x=0.$
1 Ordinary Differential Equations
18.2 The damped spring
$F= \underbrace{-\beta x'}_{\text{damping force}}\quad \underbrace{-k(s+x)}_{\text{restoring force}}+ \underbrace{m g}_{\text{gravitational force}}.$
Thanks to Newton's second law this yields the equation of motion
$m x''+\beta x'+k x=0.$
This equation only depends on the ratios of $k/m$ and $\beta/m,$ and it is customary to not only set
$\ds \omega^2=\frac{k}{m}>0\,$ but also $\,\ds 2p=\frac{\beta}{m}>0.$
1 Ordinary Differential Equations
18.2 The damped spring
Then the equation of motion for the damped spring is given by
$x''+2p\,x'+\omega^2 x=0.$
This is a homogeneous second-order linear ODE with constant coefficients.
The corresponding characteristic equation is given by
$\lambda^2+2p\lambda+\omega^2=0,$
which has discriminant
$D=4\left(p^2-\omega^2\right).$
1 Ordinary Differential Equations
18.2 The damped spring
$x''+2p\,x'+\omega^2 x=0.$
$\lambda^2+2p\lambda+\omega^2=0 \implies D=4\left(p^2-\omega^2\right).$
There are three different cases to consider.
-
$D\lt 0$ (i.e., $p\lt \omega$).
This is the underdamped or weakly-damped spring. -
$D=0$ (i.e., $p=\omega$).
This is the critically-damped spring. -
$D>0$ (i.e., $p>\omega$).
This is the overdamped or strongly-damped spring.
1 Ordinary Differential Equations
1.8.3 Underdamping
For $p\lt \omega$ the characteristic equation
$ \lambda^2+2p\lambda+\omega^2=0 $
has two complex conjugate roots
$ \lambda_1=-p+i\sqrt{\omega^2-p^2}\;\;\text{and}\;\; \lambda_2=-p-i\sqrt{\omega^2-p^2}. $
Two linearly independent solutions are thus
$e^{-p t}\cos(\omega_p t)\;\;\text{and}\;\; e^{-p t}\sin(\omega_p t),$
where
$ \omega_p=\sqrt{\omega^2-p^2}$ $=\omega\sqrt{1-(p/\omega)^2}.$
1 Ordinary Differential Equations
1.8.3 Underdamping
The general solution is
$ x(t)=e^{-p t}\Bigl(c_1\cos(\omega_p t)+c_2\sin(\omega_p t)\Bigr). $
This figure shows an example of underdamped motion:
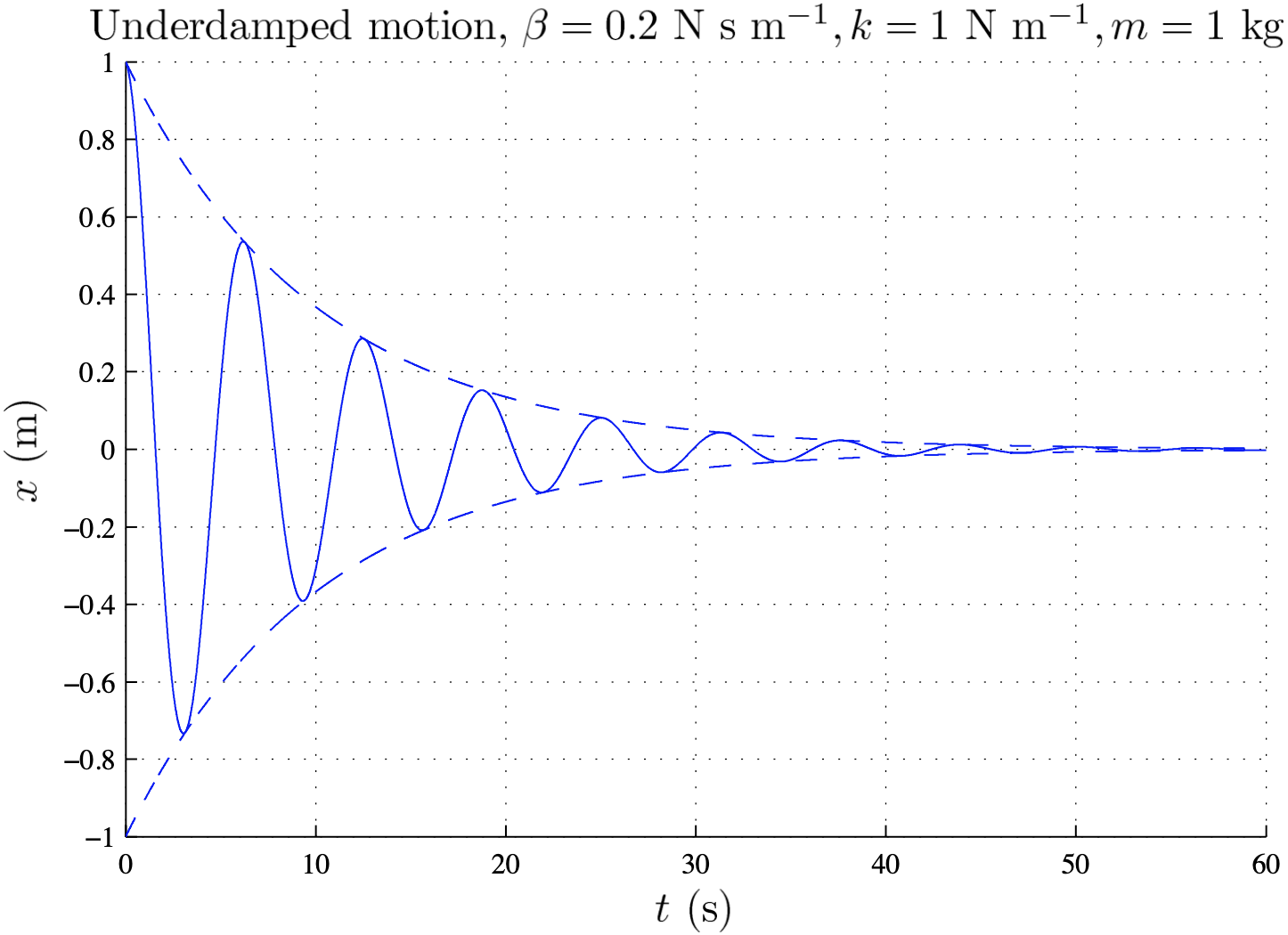
1 Ordinary Differential Equations
1.8.3 Underdamping
$ x(t)=e^{-p t}\Bigl(c_1\cos(\omega_p t)+c_2\sin(\omega_p t)\Bigr). $

1 Ordinary Differential Equations
1.8.3 Underdamping
It is best to write the general solution in terms of a single trigonometric function:
$ x(t)=Ae^{-p t}\cos(\omega_p t-\phi) $ $ =A(t)\cos(\omega_p t-\phi). $
Here $A(t)$ is a time-dependent amplitude (as shown in the above figure with the dashed lines):
$A(t)=Ae^{-p t}$
and $\omega_p$ is the frequency of the underdamped spring.
1 Ordinary Differential Equations
1.8.3 Underdamping
Since
$ \ds \frac{\omega_p}{\omega} $ $ =\sqrt{1-(p/\omega)^2} $ $ \lt 1 $
the damping of the spring leads to a frequency red-shift and an increase in the period of the spring.
When $p$ approaches $\omega$ the period tends to infinity so that we may expect that the critically-damped spring does not display oscillatory motion. This is the case considered in the next section.
1 Ordinary Differential Equations
1.8.3 Underdamping
Example: A mass of $1$kg is attached to a spring hanging under gravity with damping constant $0.2$ and spring constant $4$. Find the position of the mass after time $t$ if it is pulled down $1$m from the equilibrium position and released without kick.
Recall $\,\ds x''+ \frac{\beta}{m}x' +\frac{k}{m}x = 0$
Then $\,m = 1,$ $\,\beta = 0.2,$ $\,k = 4.$
Initial conditions: $x(0)=1,$ $\,x'(0)=0.$
1 Ordinary Differential Equations
1.8.3 Underdamping
$\ds x''+ \frac{\beta}{m}x' +\frac{k}{m}x = 0;$ $\;\,m = 1,$ $\,\beta = 0.2,$ $\,k = 4.$ IVP: $x(0)=1,$ $\,x'(0)=0.$
Thus $\;\ds x''+ 0.2x' +4x = 0. $ What is the solution $x(t)$?
Rewrite $\;\ds x''+ \dfrac{1}{5}x' +4x = 0. $
Consider $\;\ds \lambda^2+ \dfrac{1}{5}\lambda +4 = 0. $
$\ds \Ra \lambda = \frac{-\dfrac{1}{5}\pm \sqrt{\left(\dfrac{1}{5}\right)^2-4(1)(4)}}{2}$ $\ds = - \frac{1}{10} \pm \frac{\sqrt{399}}{10} i$
1 Ordinary Differential Equations
1.8.3 Underdamping
Initial conditions: $x(0)=1,$ $\,x'(0)=0.$
$\ds \Ra \lambda = - \frac{1}{10} \pm \frac{\sqrt{399}}{10} i$
General sol:
$\ds x(t) = $ $\ds e^{-\frac{1}{10} t}\left[c_1 \cos \left(\frac{\sqrt{399}}{10} t\right) + c_2 \sin \left(\frac{\sqrt{399}}{10} t\right)\right] $
Now since $\,\ds x(0) = 1 $ $\ds = c_1(1) + c_2 (0)$ $\,\ds \Ra c_1 =1.$
1 Ordinary Differential Equations
1.8.3 Underdamping
Initial conditions: $x(0)=1,$ $\,x'(0)=0.$
$\ds x(t) = $ $\ds e^{-\frac{1}{10} t}\left[c_1 \cos \left(\frac{\sqrt{399}}{10} t\right) + c_2 \sin \left(\frac{\sqrt{399}}{10} t\right)\right] $
$\,\ds \Ra c_1 =1.$
$\ds x'(t) = $ $\ds -\frac{1}{10} e^{-\frac{1}{10} t}\left[c_1 \cos \left(\frac{\sqrt{399}}{10} t\right) + c_2 \sin \left(\frac{\sqrt{399}}{10} t\right)\right] $
$\quad \;\; \ds + \, e^{-\frac{1}{10} t}\left[-\frac{\sqrt{399}}{10}c_1 \sin \left(\frac{\sqrt{399}}{10} t\right) + \frac{\sqrt{399}}{10} c_2 \cos \left(\frac{\sqrt{399}}{10} t\right)\right] $
Since $\,\ds x'(0) = 0 $ $\ds = -\dfrac{1}{10} c_1 + \frac{\sqrt{399}}{10} c_2$ $\,\ds \Ra c_2 =\frac{1}{\sqrt{399}}.$
1 Ordinary Differential Equations
1.8.3 Underdamping
Example: A mass of $1$kg is attached to a spring hanging under gravity with damping constant $0.2$ and spring constant $4$. Find the position of the mass after time $t$ if it is pulled down $1$m from the equilibrium position and released without kick.
With the initial conditions $\,x(0)=1,$ $\,x'(0)=0;$ we obtain:
$$\,\ds c_1 =1\quad \text{and}\quad \ds c_2 =\frac{1}{\sqrt{399}}.$$
Therefore
$\ds\; x(t) = e^{-\frac{1}{10} t}\left[ \cos \left(\frac{\sqrt{399}}{10} t\right) + \frac{1}{\sqrt{399}} \sin \left(\frac{\sqrt{399}}{10} t\right)\right] .$ 😀
1 Ordinary Differential Equations
1.8.3 Underdamping