Multivariate Calculus
&
Ordinary Differential Equations
Lecture 9
Super powers (analytic methods) to solve ODEs 🚀

- If $\,\ds \frac{dy}{dx}= f(x),$ then $\ds y = \int f(x) dx+C$ is the general solution.
- If $\,\ds \frac{d^2y}{dx^2}= f(x),$ then integrate twice to find the general solution.
Super powers (analytic methods) to solve ODEs 🚀

- If $\,\ds \frac{dy}{dx}= f(x),$ then $\ds y = \int f(x) dx+C$ is the general solution.
- If $\,\ds \frac{d^2y}{dx^2}= f(x),$ then integrate twice to find the general solution.
- If $\,\ds \frac{dy}{dt}= f(t)g(y),$ then it is separable and
just compute: $\,\ds \int \frac{dy}{g(y)} = \int f(t) dt.$
- If $\,\ds \frac{dy}{dt} + p(t) y = q(t),$ then find integrating factor and
just solve: $\,\ds \frac{d}{dt}\big(I y\big) = I q.$
1 Ordinary Differential Equations
1.6.2 Worked-out example: RL circuit
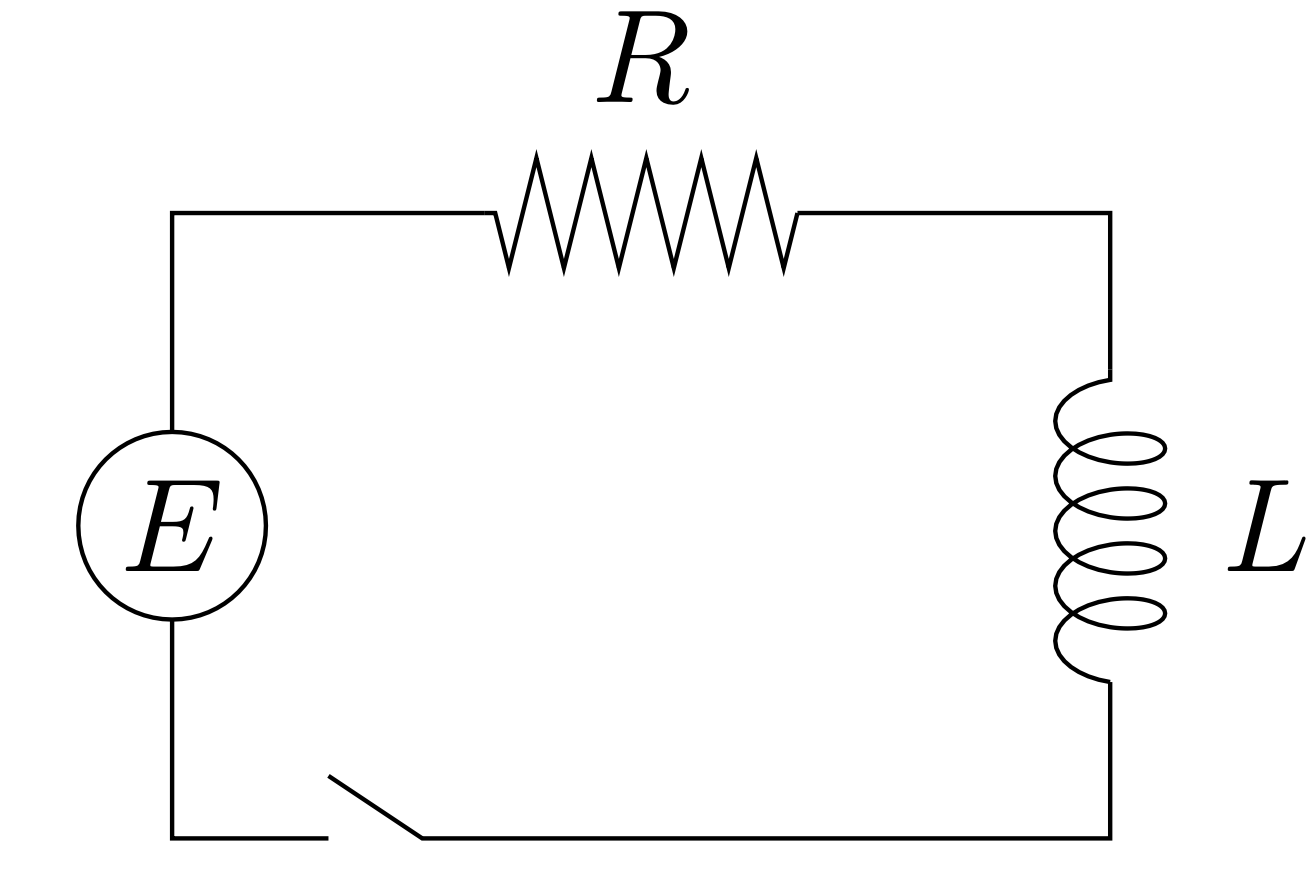
The ODE describing the current in a circuit with a resistor and an inductor (known as an RL circuit) turns out to be linear.
1 Ordinary Differential Equations
1.6.2 Worked-out example: RL circuit

To obtain this equation consider the change in voltage around the circuit. The voltage drop due to a resistor is $RJ$ where $R$ is the resistance (in Ohms) and $J$ is the current (in Amperes).
The voltage drop due to the inductor is $\ds L\dif{J}{t}$ where $L$ is the inductance (in Henries).
1 Ordinary Differential Equations
1.6.2 Worked-out example: RL circuit

To obtain this equation consider the change in voltage around the circuit. The voltage drop due to a resistor is $RJ$ where $R$ is the resistance (in Ohms) and $J$ is the current (in Amperes).
The voltage drop due to the inductor is $\ds L\dif{J}{t}$ where $L$ is the inductance (in Henries).
According to Kirchhoff's Law the sum of the voltage drops is equal to the supplied voltage $E(t)$ (in Volts). Hence
$\ds L\dif{J}{t}+RJ=E(t). \qquad (*)$
1 Ordinary Differential Equations
1.6.2 Worked-out example: RL circuit
$\ds L\dif{J}{t}+RJ=E(t). \qquad (*)$
Example: Suppose in an RL circuit a battery supplies a constant voltage of 80V, the inductance is 2H and the resistance is $10\Omega$. (i) Find an expression for $J(t),$ and (ii) determine the the current after 1 second if $J(0)=0.$
Solution: We first put $(*)$ into standard form:
$\ds\dif{J}{t}+\frac{RJ}{L}=\frac{E(t)}{L}.$
1 Ordinary Differential Equations
1.6.2 Worked-out example: RL circuit
Example: Suppose in an RL circuit a battery supplies a constant voltage of 80V, the inductance is 2H and the resistance is $10\Omega$. (i) Find an expression for $J(t),$ and (ii) determine the the current after 1 second if $J(0)=0.$
Solution: We first put $(*)$ into standard form:
$\ds\dif{J}{t}+\frac{RJ}{L}=\frac{E(t)}{L}.$
An integrating factor for this ODE is
$\ds I(t)=\exp\left(\frac{R}{L} \int \dup t\right)$ $\ds = \exp\left(\frac{Rt}{L}\right)$ $\ds = {\large e^{Rt/L}}.$
1 Ordinary Differential Equations
1.6.2 Worked-out example: RL circuit
Example: Suppose in an RL circuit a battery supplies a constant voltage of 80V, the inductance is 2H and the resistance is $10\Omega$. (i) Find an expression for $J(t),$ and (ii) determine the the current after 1 second if $J(0)=0.$
Solution: Multiplying the ODE $\,\ds\dif{J}{t}+\frac{RJ}{L}=\frac{E(t)}{L}\,$ by this factor and using the product rule gives
$\ds {\large e^{Rt/L}}\dif{J}{t}+{\large e^{Rt/L}}\frac{RJ}{L}=\frac{E(t)}{L}{\large e^{Rt/L}}$
$\ds \Ra \dif{}{t}\Bigl({\large e^{Rt/L}}J\Bigr)=\frac{E(t)}{L}{\large e^{Rt/L}}$
1 Ordinary Differential Equations
1.6.2 Worked-out example: RL circuit
Example: Suppose in an RL circuit a battery supplies a constant voltage of 80V, the inductance is 2H and the resistance is $10\Omega$. (i) Find an expression for $J(t),$ and (ii) determine the the current after 1 second if $J(0)=0.$
Solution:
$\ds \Ra \dif{}{t}\Bigl({\large e^{Rt/L}}J\Bigr)=\frac{E(t)}{L}{\large e^{Rt/L}}$
Integrating the previous equation leads to
$\ds e^{Rt/L}J=\frac{1}{L}\int E(t) e^{Rt/L}\dup t$
$\Ra \ds J(t)=\frac{1}{L}e^{-Rt/L} \int E(t) e^{Rt/L}\dup t.$
1 Ordinary Differential Equations
1.6.2 Worked-out example: RL circuit
Example: Suppose in an RL circuit a battery supplies a constant voltage of 80V, the inductance is 2H and the resistance is $10\Omega$. (i) Find an expression for $J(t),$ and (ii) determine the the current after 1 second if $J(0)=0.$
Solution:
$\Ra \ds J(t)=\frac{1}{L}e^{-Rt/L} \int E(t) e^{Rt/L}\dup t.$
So far we have treated everything completely generally. Now we use that the battery supplies constant voltage, i.e., $E$ does not depend on $t$. This yields
$\ds J(t)=\frac{E}{L}e^{-Rt/L} \int e^{Rt/L}\dup t $
1 Ordinary Differential Equations
1.6.2 Worked-out example: RL circuit
Example: Suppose in an RL circuit a battery supplies a constant voltage of 80V, the inductance is 2H and the resistance is $10\Omega$. (i) Find an expression for $J(t),$ and (ii) determine the the current after 1 second if $J(0)=0.$
Solution:
$\ds J(t)=\frac{E}{L}e^{-Rt/L} \int e^{Rt/L}\dup t $
$\Ra \ds J(t)=\frac{E}{L}e^{-Rt/L} \Bigl(\frac{L}{R}e^{Rt/L}+C\Bigr),$
so that we finally obtain
$\Ra \ds J(t)=\frac{E}{R}+D e^{-Rt/L}.$
1 Ordinary Differential Equations
1.6.2 Worked-out example: RL circuit
Example: Suppose in an RL circuit a battery supplies a constant voltage of 80V, the inductance is 2H and the resistance is $10\Omega$. (i) Find an expression for $J(t),$ and (ii) determine the the current after 1 second if $J(0)=0.$
Solution:
$\Ra \ds J(t)=\frac{E}{R}+D e^{-Rt/L}.$
Since $J(0)=0,$ the constant $D=-E/R:$
$\Ra \ds J(t)=\frac{E}{R}\Bigl(1-e^{-Rt/L}\Bigr).$
1 Ordinary Differential Equations
1.6.2 Worked-out example: RL circuit
Example: Suppose in an RL circuit a battery supplies a constant voltage of 80V, the inductance is 2H and the resistance is $10\Omega$. (i) Find an expression for $J(t),$ and (ii) determine the the current after 1 second if $J(0)=0.$
Solution:
$\Ra \ds J(t)=\frac{E}{R}\Bigl(1-e^{-Rt/L}\Bigr).$
Since $E=80,$ $R=10$ and $L=2:$
$\Ra \ds J(t)=8\Bigl(1-e^{-5t}\Bigr).$
We can finally determine $\,J(1)\,$ as $\,J(1)=8(1-\exp(-5))\,$ Amperes.
1 Ordinary Differential Equations
1.6.2 Worked-out example: RL circuit
Remember that we derived a completely general solution to the ODE for any applied voltage $E(t)$:
$\ds J(t)=\frac{1}{L}e^{-Rt/L} \int E(t) e^{Rt/L}\dup t.$
So if we had a non-constant voltage such as $$E(t)=E_0\sin(\omega t),$$ we would be able to find the solution for this case by evaluating the integral.
1 Ordinary Differential Equations
1.6.3 Main points
- You need to be able to identify a first-order linear ODE.
- You need to be able to solve a first-order linear ODE using an integrating factor.